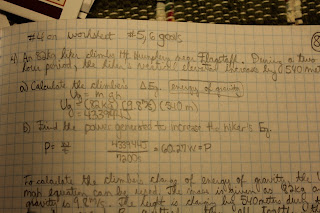A candle with a flame 1.5cm tall is placed 5.0cm from the front of a concave mirror. A virtual image is produced that is 10cm from the vertex of the mirror. (a) Find the focal length and radius of curvature of the mirror (b) How tall is the image of the flame?
Sunday, June 2, 2013
Energy Problem (The Hiker)
To calculate the climber's change of energy of gravity, the Ug=mgh equation can be used. The mass is given as 82kg and gravity is 9.8m/s. The height is changing by 540meters during the two hour period. By multiplying them all together, the change in energy due to gravity is 433944Joules.
The second part of the question asks to find the power needed to increase the hikers Eg. Here, the power equation can be used which is Power equals work divided by time. The work is the change in energy just calculated, 433944Joules. The time is 2hours which needs to be converted to seconds to be calculated properly. One hour contains 3600seconds. Therefore, 3600seconds multiplied by 2hours equals a time of 7200seconds. Finally to find the power, take the work, 433944Joules, divided by the time in seconds, 7200seconds, to find the power generated which is 60.27W. 60.27W represents the power generated to increase the hiker's Eg.
The second part of the question asks to find the power needed to increase the hikers Eg. Here, the power equation can be used which is Power equals work divided by time. The work is the change in energy just calculated, 433944Joules. The time is 2hours which needs to be converted to seconds to be calculated properly. One hour contains 3600seconds. Therefore, 3600seconds multiplied by 2hours equals a time of 7200seconds. Finally to find the power, take the work, 433944Joules, divided by the time in seconds, 7200seconds, to find the power generated which is 60.27W. 60.27W represents the power generated to increase the hiker's Eg.
Energy Problem (The Roller Coaster)
 A roller coaster travels on a frictionless track as shown in Fig 5.31. (a) If the speed of the roller coaster at point A is 5.0m/s, what is its speed at point B? (b) Will it reach point C? (c) What minimum speed at point A is required for the roller coaster to reach point C?
A roller coaster travels on a frictionless track as shown in Fig 5.31. (a) If the speed of the roller coaster at point A is 5.0m/s, what is its speed at point B? (b) Will it reach point C? (c) What minimum speed at point A is required for the roller coaster to reach point C? To create the bar graphs, the knowledge of what K, Ug, and Us is in order. While Ug represents the height of the object, K symbolizes the potential energy and Us represents a spring energy. During point A, the initial energy graph needs an equal amount of K and Ug. This is because the roller coaster is 5.0meters off the ground and therefore has the potential energy, Ug, to go down the hill. There is no spring throughout this ride. At point B, the intermediate energy the height, Ug, is gone and transferred to potential energy, K. Since the roller coaster started out with 5.0m/s velocity and at the top of a hill, the coaster now needs all the energy it lost from its height, Ug, in potential energy to make it up the second hill. By point C, the final energy graph represents the transfer of all the energy to Ug, height. The coaster is now at 8.0 meters in height, which is taller than the first hill. The potential energy it had at points A and B were used to propel the roller coaster to point C.
To create the bar graphs, the knowledge of what K, Ug, and Us is in order. While Ug represents the height of the object, K symbolizes the potential energy and Us represents a spring energy. During point A, the initial energy graph needs an equal amount of K and Ug. This is because the roller coaster is 5.0meters off the ground and therefore has the potential energy, Ug, to go down the hill. There is no spring throughout this ride. At point B, the intermediate energy the height, Ug, is gone and transferred to potential energy, K. Since the roller coaster started out with 5.0m/s velocity and at the top of a hill, the coaster now needs all the energy it lost from its height, Ug, in potential energy to make it up the second hill. By point C, the final energy graph represents the transfer of all the energy to Ug, height. The coaster is now at 8.0 meters in height, which is taller than the first hill. The potential energy it had at points A and B were used to propel the roller coaster to point C. The speed at point B can be calculated with the equations K, potential energy, equals 1/2 mass multiplied by velocity squared and Ug, height, equals mass multiplied by gravity, 9.8m/s, and height, in meters. Since the mass of the coaster isn't given, the combination of the equations need to be used to find the speed at point B. If 1/2 mass times velocity squared plus mass times gravity times height is put equal to 1/2 mass times velocity at point B squared, the velocity can be determined. Because the mass isn't given, one can begin by dividing the entire equation by mass. Then, plug in the numbers-1/2 (5m/s) squared + (9.8m/s) (5m) equals 1/2 B's velocity squared. B's velocity is then found to be 11.09m/s.
The speed at point B can be calculated with the equations K, potential energy, equals 1/2 mass multiplied by velocity squared and Ug, height, equals mass multiplied by gravity, 9.8m/s, and height, in meters. Since the mass of the coaster isn't given, the combination of the equations need to be used to find the speed at point B. If 1/2 mass times velocity squared plus mass times gravity times height is put equal to 1/2 mass times velocity at point B squared, the velocity can be determined. Because the mass isn't given, one can begin by dividing the entire equation by mass. Then, plug in the numbers-1/2 (5m/s) squared + (9.8m/s) (5m) equals 1/2 B's velocity squared. B's velocity is then found to be 11.09m/s.To find if the coaster reaches point C, the right side of the equation above is used equaled to the height equation, Ug equals gravity times height times mass. Again, divide the entire equation by mass. Plug in the numbers, 1/2 (5m/s) squared + (9.8m/s) (5m) equals (9.8m/s) times height. By solving for height, the height comes out to be 6.28m. The coaster doesn't reach point C at 8meters.
Finally to locate the minimum speed, velocity, required to reach point C from point A, use the equation from the last paragraph keeping the velocity as the variable, 1/2(v) squared + (9.8) (5m) ='s (9.8) (8). The height of A is used in the first half of the equation because the coaster starts here and ends at 8meters. That's why 8meters is used in the second half of the equation. The velocity needed at point A to reach 8meters at point C is 7.67m/s.
Subscribe to:
Comments (Atom)